From Wikipedia, the free encyclopedia
Trägheitsmoment für ein massives, homogenes Ellipsoid Für eine Rotation um die x-Achse ist das Trägheitsmoment:
I
x
=
∫
V
(
y
2
+
z
2
)
ρ
d
V
=
∫
V
y
2
ρ
d
V
+
∫
V
z
2
ρ
d
V
{\displaystyle I_{x}=\int _{V}(y^{2}+z^{2})\rho dV=\int _{V}y^{2}\rho dV+\int _{V}z^{2}\rho dV}
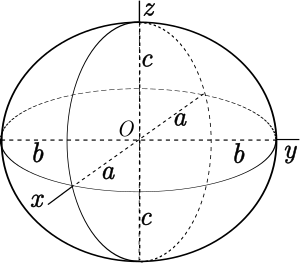
Ellipsoid der Masse m mit gleichmäßiger Dichte und Form beschrieben durch
(
x
/
a
)
2
+
(
y
/
b
)
2
+
(
z
/
c
)
2
≤
1
{\displaystyle (x/a)^{2}+(y/b)^{2}+(z/c)^{2}\leq 1}
(
I
x
,
I
y
,
I
z
)
=
(
b
2
+
c
2
,
a
2
+
c
2
,
a
2
+
b
2
)
⋅
m
/
5
{\displaystyle (I_{x},I_{y},I_{z})=(b^{2}+c^{2},a^{2}+c^{2},a^{2}+b^{2})\cdot m/5\,}
Setze
u
=
x
/
a
,
v
=
y
/
b
,
w
=
z
/
c
,
{\displaystyle u=x/a,\,v=y/b,\,w=z/c,\,}
∫
V
z
2
ρ
d
V
=
ρ
⋅
a
b
c
⋅
c
2
⋅
K
,
{\displaystyle \int _{V}z^{2}\rho dV=\rho \cdot abc\cdot c^{2}\cdot K,\,}
K
=
∫
u
=
−
1
1
∫
v
=
−
1
−
u
2
1
−
u
2
∫
w
=
−
1
−
u
2
−
v
2
1
−
u
2
−
v
2
w
2
d
u
d
v
d
w
,
{\displaystyle K=\int _{u=-1}^{1}\int _{v=-{\sqrt {1-u^{2}}}}^{\sqrt {1-u^{2}}}\int _{w=-{\sqrt {1-u^{2}-v^{2}}}}^{\sqrt {1-u^{2}-v^{2}}}w^{2}dudvdw,\,}
∫
V
y
2
ρ
d
V
=
ρ
⋅
a
b
c
⋅
b
2
⋅
K
,
{\displaystyle \int _{V}y^{2}\rho dV=\rho \cdot abc\cdot b^{2}\cdot K,\,}
I
x
=
ρ
⋅
a
b
c
⋅
(
c
2
+
b
2
)
⋅
K
=
3
m
4
π
(
c
2
+
b
2
)
⋅
K
,
{\displaystyle I_{x}=\rho \cdot abc\cdot (c^{2}+b^{2})\cdot K={\frac {3m}{4\pi }}(c^{2}+b^{2})\cdot K,\,}
m
=
ρ
⋅
V
=
ρ
⋅
4
π
3
⋅
a
b
c
{\displaystyle m=\rho \cdot V=\rho \cdot {\frac {4\pi }{3}}\cdot abc}
Eine massive Kugel mit a=b=c hat
I
x
=
3
m
4
π
⋅
2
a
2
⋅
K
{\displaystyle I_{x}={\frac {3m}{4\pi }}\cdot 2a^{2}\cdot K}
Trägheitsmoment (Rotoationsachse durch den Mittelpunkt) von
I
x
=
2
5
m
a
2
{\displaystyle I_{x}={\frac {2}{5}}ma^{2}}
K
=
4
π
/
15
{\displaystyle K=4\pi /15}
Also:
I
x
=
m
5
(
b
2
+
c
2
)
{\displaystyle I_{x}={\frac {m}{5}}(b^{2}+c^{2})\,}