Talk:Small complex icosidodecahedron
| This article is rated Stub-class on Wikipedia's content assessment scale. It is of interest to the following WikiProjects: | |||
| |||
Vertex figure(s)
[edit]What would a true vertex figure look like? 4 T C 12:47, 25 January 2010 (UTC)
 - It's the union of the verfs of two polyhedra. (Label pentagram edges as 5, and pentagon edges as 3) Tom Ruen (talk) 21:39, 25 January 2010 (UTC)
- It's the union of the verfs of two polyhedra. (Label pentagram edges as 5, and pentagon edges as 3) Tom Ruen (talk) 21:39, 25 January 2010 (UTC)
Email reply from Richard Klitzing:
Cid "looks" like a compound of ike and gad. But there are several thingies with that shape to be distinguished. Look first at the vertex figure. Someone called that a "complete" pentagon. In fact it can be given A) as [3,3,3,3,3] + [5,5,5,5,5]/2. Likewise B) as [3,5,3,5,3,5,3,5,3,5]/3 (both prograde) or C) as [3/2,5,3/2,5,3/2,5,3/2,5,3/2,5] (alternating prograde and retrograde sides). While A) is the compound, B) and C) are (different) true polytopes, cause they do not fall apart. Nevertheless they are degenerate, as they have coincident edges. Any icosahedral edge will be doubled, independing of interpretation A-C). But while A) also doubles the vertices, B) and C) just has 12 vertices, each with 10 emanating edges. - The paper you cited [1] is the right one.
You might read a bit on that topic in the realm of compounds, [2] giving there some other examples too.
These generate forms are listed in Coxeter's 1954 paper, copied at[3] (Table 6, degenerate cases) This polyhedron is both the second and forth vertex figure listed. Tom Ruen (talk) 22:16, 25 January 2010 (UTC)
- I linked the two CORRECT vertex figures (TWO of them) from Coxeter's paper. Tom Ruen (talk) 02:23, 26 January 2010 (UTC)
 .
.
truncated great stellated dodecahedron
[edit]I removed this claim, don't think it's true: It occurs as the truncated great stellated dodecahedron.
At Wythoff_symbol#Overlapping_spherical_tilings_.28r.3D2.29, the truncated great stellated dodecahedron is a degenerate uniform polyhedra with vertex figure 3.10/2.10/2. It might LOOK the same, but then we have a THIRD vertex figure for this geometry. Tom Ruen (talk) 02:52, 26 January 2010 (UTC)
Which looks like this:

4 T C 08:34, 26 January 2010 (UTC)
Here are a full set of 9 vertex figures (from the 1954 paper) that apparently create this same degenerate geometry. He lists the Wythoff symbols are given AND the compound coverings. Tom Ruen (talk) 00:15, 29 January 2010 (UTC)
Yup, truncated gissid is not cid: it is 2gad+ike. (Similar except there are two coincident great dodecahedra and one icosahedron sharing the same edges and vertices.) Double sharp (talk) 13:07, 22 April 2014 (UTC)
language needs work
[edit]- The star has 32 faces (20 triangles and 12 pentagons), 60 (doubled) edges and 12 vertices and 4 sharing faces. The faces in it are considered as two overlapping edges as topological polyhedron.
What does "4 sharing faces" mean? What does the last sentence mean?
Either it has 60 edges (coinciding in pairs) or it has 30 edges each belonging to four faces. Don't conflate the two interpretations.
Hm. For each edge (or double-edge) there are three ways to pair the faces. How many topologically distinct surfaces are formed by such pairings? —Tamfang (talk) 23:01, 22 April 2014 (UTC)
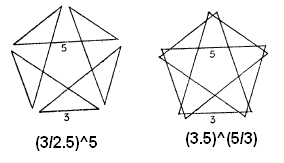
- I see at least 4 different interpretations of cid, and the article should probably not take any of them as the standard one. You could split the edges alone. Then you can have (A) all the faces prograde (vertex configuration (3.5)5/3), or (B) have one set of faces retrograde and the other prograde (vertex configuration (3/2.5)5). Or you could (C) not split the edges and have four faces incident at each edge. There is additionally the option of (D) splitting the edges and vertices and treating this as a compound polyhedron. Gacid (the great complex icosidodecahedron) similarly also has at least 4 interpretations. Double sharp (talk) 15:04, 23 April 2014 (UTC)
Broken Link at the bottom of the page
[edit]3 of the 4 links in further reading all link to the same page; being Small complex rhombicosidodecahedron — Preceding unsigned comment added by OmarRihani7777777 (talk • contribs) 00:43, 9 May 2022 (UTC)


