Talk:Levi-Civita symbol/Archive 1

Archive 1

Examples

Could someone please explain the examples more clearly. Phrases like "it is obvious that.." or "it is clear that..." are hot very helpful, as it is all relative. Could someone please outline the steps for example 2 (and others if necessary) clearly, to be like an example is meant to be.

Levi-Civita (person)

Was Levi-Civita really a physician? I see nothing on that on his biographical article.Commander Nemet 05:25, 15 March 2006 (UTC)

Clearly this person means physicist. I have altered the article appropriately.Roonilwazlib 19:07, 22 May 2006 (UTC)

" It is actually a pseudotensor because under an orthogonal transformation of jacobian determinant −1 (i.e., a rotation composed with a reflection), it gets a -1." - It gets a -1? Could someone clarify this, maybe writing it explicitly with symbols? I don't get it. I'm trying to find out how the Levi-Civita symbol transforms and this hasn't helped... 203.97.255.167 22:01, 24 June 2006 (UTC)

Levi Civita is a HOLOR not a TENSOR, it does not transform like tensors do. Seeing as Wikipedia has no articles on Holor theory this may get confusing. Also the index conventions on this page are all wrong "superscipts should be considered equivalent with subscripts". Wrong.

I don't know what is a Holor. But they were talking about "The tensor whose components in an orthonormal basis are given by the Levi-Civita symbol" and it is tensor. Gets -1 means if the basis of underlying vector space is changed by matrix A=diag(-1,-1,-1) then components of permutation tensor are changed according to $\varepsilon '_{ijk}=A_{im}A_{jn}A_{kl}\varepsilon _{mnl}=-\varepsilon _{ijk}$ but it is normal behaviour of tensors and it does not mean that this permutation tensor is pseudotensor. The problem with so called pseudotensor is related to orientation of the underlying vector space. —Preceding unsigned comment added by Matarife.cz (talk • contribs) 20:18, 23 September 2008 (UTC)

The Levi-Civita symbol is not an ordinary tensor, it is a tensor density. In other words, its rule for transforming when one changes coordinate systems includes a factor which is a (nontrivial) power of the Jacobian of the coordinate transformation in addition to the usual factors which appear in coordinate transformations of tensors. JRSpriggs (talk) 05:16, 24 September 2008 (UTC)

Visualization matrix for $\epsilon _{ijk}$

I think the image showing the visualization of the symbol is not correct. If i corresponds to row, j corresponds to column, and k corresponds to the plane, then one should have the following representation:

first plane:

     0     0     0

     0     0     1

     0    -1     0

second plane:

     0     0    -1

     0     0     0

     1     0     0

third plane:

     0     1     0

    -1     0     0

     0     0     0

I may be wrong if i,j and k correspond to different directions than the one I mentioned above. Please correct. -- Myth (Talk) 14:17, 18 March 2007 (UTC)

I second this. They are obviously wrong, as the formulaic representation within the text states: e123=1 while the picture shows a -1. I also get the solution given above. How can the image be changed? -- User:xmaster1123 —Preceding comment was added at 08:58, 6 November 2007 (UTC)

Of course the sum is n!

The article asserts that it can be shown that (in n dimensions) the sum of the symbol as all its indices vary from 1 to n is n! Well, yeah! The symbol is nonzero precisely when its square is 1, which is precisely when the indices are a permutation of [n]. So the sum is exactly a count of the permutations of an n-set. And how many permutations exist? Why n! of them. QED.

My point is that the claim that "it can be shown..." strikes me as rather modest, since the proof is nearly self-evident. Anybody disagree?—PaulTanenbaum (talk) 03:48, 15 January 2008 (UTC)

error

There is a conflict between the 2 different places where the formula for the product of two LC symbols with 3 indices with 1 contracted appear. —Preceding unsigned comment added by 74.79.30.13 (talk) 22:44, 17 February 2008 (UTC)

Other notation for the three dimensional Levi-Civita symbol without case differentiation

$\varepsilon _{ijk}={\frac {\left(j-i\right)\left(k-j\right)\left(k-i\right)}{2}}$

--77.125.156.157 (talk) 13:46, 28 May 2008 (UTC)

Question: plus or minus in 4 dimensions?

I learned somewhere else (e.g. Jackson's Classical Electrodynamics) that ε^αβγδ= - ε_αβγδ, which has a minus sign.
Should here be a minus sign or not? (See the formula in section "Tensor density") —Preceding unsigned comment added by 129.125.101.226 (talk • contribs)

No. Why would there be a minus sign? It would just cause confusion. JRSpriggs (talk) 01:14, 24 October 2008 (UTC)

I think there should be. Confusion doesn't come into it - can someone with a bit more experience than myself check this? I guess it's a question of raising the indices 4 times, and seeing what the result is. Incidentally, the problem set I'm doing at the moment requires it to be negative to get the right answer, but obviously that's not conclusive... (Tomhosking (talk) 22:12, 10 January 2009 (UTC))

Yup, there should be. Taking $\epsilon _{ijkl}=\pm 1$ for even/odd permutations of ijkl as usual, and a standard metric signature (+---) or (-+++), we can find $\epsilon ^{ijkl}$:

$\epsilon ^{abcd}=g^{ai}g^{bj}g^{ck}g^{dl}\epsilon _{ijkl}$

= 0 unless a=i, b=j, etc.</math>

Let's take abcd = 1234 as an example:

$\epsilon ^{1234}=g^{1i}g^{2j}g^{3k}g^{4l}\epsilon _{ijkl}$

$=g^{11}g^{22}g^{33}g^{44}\epsilon _{1234}$

$=1\times -1\times -1\times -1\times \epsilon _{1234}$

$=-1\times \epsilon _{1234}$

And equivalently for a (-+++) metric

But since no two metric values will be repeated (as $\epsilon _{ijkl}$ would equal 0), there will always be an odd number of minus signs in the product of the metrics (no matter which signature is used) - ie, this is true for all ijkl, and thus:

$\epsilon ^{ijkl}=-\epsilon _{ijkl}~(nosum)$

Given that the only time you work in 4D is with a metric (+---) or (-+++) (unless it's GR, but that's unnecessarily complicated here) then my edit was correct, so I'll change it back, and make it explicit which metric is being used. Tomhosking (talk) 17:47, 27 January 2009 (UTC)

To Tomhosking: Your error is in your basic premise, "it's a question of raising the indices 4 times", which is false in this case. All the versions of the symbol which are referred to in the section Levi-Civita symbol#Tensor density are intended to be mathematically identical (with all components being either 0, +1, or -1 in any coordinate system) and independent of the metric. Just as $\delta _{\beta }^{\alpha }$ is independent of the metric. Otherwise, they would not be tensor densities as described. In other words, you are confusing it with an ordinary tensor which is defined originally only in its covariant form; and then extended to a contravariant form by raising indices. JRSpriggs (talk) 06:20, 28 January 2009 (UTC)

To Tomhosking: I think your argument is right. Misner Thorne and Wheeler do as well; see page 87. -Guest —Preceding unsigned comment added by 138.16.76.11 (talk) 04:17, 25 March 2010 (UTC)

The issue seems to be resolved by section 'Ordinary tensor', but absence of explicitly stated formula in case of special relativity still can confuse reader. So I've took the liberty of adding it as an example to 'Ordinary tensor' section. —Preceding unsigned comment added by 94.179.189.134 (talk) 02:17, 10 November 2010 (UTC)

Not according to Griffiths 1987 p239. 82.139.86.96 (talk) 02:59, 17 December 2009 (UTC)

Eddington Epsilon

In Geometric Computation For Machine Vision by Kanatani, p 116 footnote calls this the Eddington epsilon). —Ben FrantzDale (talk) 17:05, 20 November 2008 (UTC)

Error in use of Einstein convention

Einstein convention requires the repeated summation index to be upper and lower. So

$(A\times B)^{i}=\varepsilon ^{ijk}A^{j}B^{k}$

should be written

$(A\times B)_{i}=\varepsilon _{ijk}A^{j}B^{k}.$

This error was copied from Planet Math.

Bo Jacoby (talk) 20:30, 23 January 2009 (UTC).

To Bo Jacoby: If one chooses a Cartesian coordinate system for three dimensional space (signature +++), then the metric tensor is $g_{ij}=\delta _{ij}$. Consequently, in such coordinate systems, there is no effective difference between covariant and contravariant indices. However, the cross product of two (ordinary) vectors is an axial vector which may be regarded as a vector density (tensor density) since the Jacobian determinant of some transformations may be -1 rather than +1. JRSpriggs (talk) 03:15, 24 January 2009 (UTC)

So you're saying that the current example holds only for a specific metric, and not in general? In which case, why not change it to hold for all metrics? Tomhosking (talk) 13:59, 25 January 2009 (UTC)

Thank you. But why not use a notation which is valid also when there is an effective difference between covariant and contravariant indices? Bo Jacoby (talk) 08:18, 25 January 2009 (UTC).

How would one interpret $A\times (B\times C)$, if we use Bo's suggestion? JRSpriggs (talk) 13:38, 26 January 2009 (UTC)

Like this, I guess:

$(A\times (B\times C))_{i}=\varepsilon _{ijk}\varepsilon ^{kmn}A^{j}B_{m}C_{n}$$=(\delta _{j}^{m}\delta _{i}^{n}-\delta _{j}^{n}\delta _{i}^{m})A^{j}B_{m}C_{n}$$=(A^{j}B_{j})C_{i}-(A^{j}C_{j})B_{i}\,$$=((A\cdot B)C-(A\cdot C)B)_{i}\,$,

Bo Jacoby (talk) 00:07, 29 January 2009 (UTC).

To Bo Jacoby: I think that you have the sign reversed; see Triple product#Vector triple product.

It seems that your choice of which indices are covariant and which are contravariant is somewhat arbitrary. Would it not be better to introduce the metric explicitly, getting something like $g^{hi}\varepsilon _{ijk}A^{j}g^{kl}\varepsilon _{lmn}B^{m}C^{n}\,$? JRSpriggs (talk) 09:38, 29 January 2009 (UTC)

That would be a clutter without a good reason. Since inner product is invariant, who gives a damn how exactly it's written? — Kallikanzarid (talk) 15:54, 5 August 2009 (UTC)

To Kallikanzarid: In the Minkowski metric, using the metric to raise or lower an index may cause a sign change. It is not as nice as the delta. JRSpriggs (talk) 03:49, 6 August 2009 (UTC)

Why Levi-Civita symbol

Better is Levi-Civitatensor. It is really of 3rd order tensor. How you can multiply (or do other math operation) by symbol? This symbol is epsilon, but Levi-Civita is tensor (using epsilon as symbol) —Preceding unsigned comment added by 212.5.210.202 (talk) 09:35, 2 September 2009 (UTC)

I believe the answer is that it does not transform either covariantly or contravariantly. Similarly Christoffel symbols don't transform as tensors. —Ben FrantzDale (talk) 13:54, 30 November 2009 (UTC)

Incorrect Definition

Unfortunately, the definition of the Levi-Civita Tensor is incorrect.

All 3-cycles are even permutations (check this - it is quite straightforward to prove). More generally, we have the following theorem in algebra:

A m-cycle is ODD if and only if m is EVEN. (c.f. Dummit and Foote, Abstract Algebra, p.109 ff.)

Therefore, in no case should the entry -1 (when the 3-tuple is as listed) be called an "odd permutation". This is false.

The generalization on this page to multiple dimensions suffers from the same defect.

Regards, —Preceding unsigned comment added by 128.12.248.142 (talk) 05:35, 29 November 2009 (UTC)

I believe that you have been misled by an unfortunate ambiguity of notation. When the article talks about "(i,j,k) is (1,3,2)" for example, what it means is "i=1 and j=3 and k=2". It is not using the notation for cycles described at Permutation#Notation. JRSpriggs (talk) 01:59, 1 December 2009 (UTC)

I have not been misled. Your definition using tuples is correct.

However, in the paragraph immediately following the definition, we find the explanation:

"i.e. \varepsilon_{ijk} is 1 if (i, j, k) is an even permutation of (1,2,3), −1 if it is an odd permutation,"

Similarly, in the section labeled "Generalization to N-Dimensions":

"Thus, it is the sign of the permutation in the case of a permutation, and zero otherwise."

etc., etc.,.

These assertions are false and should be removed.

Regards, —Preceding unsigned comment added by 128.12.248.94 (talk) 05:54, 11 December 2009 (UTC)

Then I do not understand what you are saying. Those statements are correct, not erroneous. JRSpriggs (talk) 00:02, 12 December 2009 (UTC)

I will try to be more explicit then:

We find on the line immediately following the definition on this page the statement:

"E_ijk is 1 if (i,j,k) is an EVEN PERMUTATION of (1,2,3), -1 if it is an ODD PERMUTATION" (***) (author's emphasis).

Suppose this is true, for contradiction. Then since every permutation of the form (i,j,k) is even it follows that the

Levi-Civita tensor takes on the value 1 for any combination (i,j,k). This is false, whence the statement above, (***), is

false as well.

My question is this: the definition of the Levi-Civita has nothing whatsoever to do with even and odd permutations.

Why, then, can I find the words "even permutation" and "odd permutation" following said definition?

Regards, —Preceding unsigned comment added by 128.12.248.142 (talk) 01:54, 12 December 2009 (UTC)

You are still interpreting the symbol (i,j,k) incorrectly. For example, (1,3,2) in the meaning of this article refers to a permutation which takes 1->1, 2->3, 3->2. It would be called (2,3) in the notation of the article on permutations. This is an odd permutation. Thus the value is $\varepsilon _{132}=-1\,.$ JRSpriggs (talk) 01:21, 14 December 2009 (UTC)

Yes I see. I have never seen this notation before. To my knowledge, cycle notation is standard for permutations. Regards, —Preceding unsigned comment added by 84.227.177.174 (talk) 12:54, 14 December 2009 (UTC)

We are forced to do it this way because of the conventional meaning of tensor indices. JRSpriggs (talk) 17:00, 14 December 2009 (UTC)

What kind of product?

In the generalization of the symbol to an nth-order tensor, the following equation is given:

$\varepsilon _{a_{1}a_{2}a_{3}\ldots a_{n}}=\prod _{i=1}^{n-1}\left({\frac {1}{i!}}\ \prod _{j=i+1}^{n}(a_{j}-a_{i})\right)$

My question is, what kind of product do these represent? If I understand the equation correctly, it would be a tensor product. If not... well, I'm completely lost. LokiClock (talk) 03:32, 28 January 2010 (UTC)

For any particular choice of a₁ thru a_n, this equations gives the real value of a single component of the tensor. The product is an ordinary multiplication of real numbers. Perhaps it would have been better if written as

$\varepsilon _{a_{1}a_{2}a_{3}\ldots a_{n}}=\prod _{i=1}^{n-1}\prod _{j=i+1}^{n}\operatorname {sgn}(a_{j}-a_{i})$

where sgn is the sign of the number, i.e. +1 if positive, -1 if negative, 0 otherwise. Thus for example, when n=3

$\varepsilon _{213}=(\operatorname {sgn}(1-2)\operatorname {sgn}(3-2))(\operatorname {sgn}(3-1))=(-1\cdot +1)\cdot +1=-1\,.$

OK? JRSpriggs (talk) 13:42, 28 January 2010 (UTC)

Okay, that makes so much more sense. I had this strange idea about an abuse of notation with a₁, for example, being the first component of a tensor, a, used as input. I may need to play around with the equation I was thinking of anyway, though. LokiClock (talk) 05:13, 29 January 2010 (UTC)

Equation 8 and anti-symmetrization

On 18 August 2010, 188.158.105.98 (talk · contribs) again incorrectly altered equation 8 of section Levi-Civita symbol#Properties, saying "if 8 equation has (n-m)! then 6 equation will be wrong! cause (3-1)!=2 and 6 equation has not that!" in his edit summary.
Equation 6 says:

$\varepsilon _{ijk}\varepsilon ^{imn}=\delta _{j}^{m}\delta _{k}^{n}-\delta _{j}^{n}\delta _{k}^{m}\,.$

Equation 8 previously said:

$\varepsilon _{i_{1}\dots i_{k}~i_{k+1}\dots i_{n}}\varepsilon ^{i\dots i_{k}~j_{k+1}\dots j_{n}}=k!(n-k)!~\delta _{[i_{k+1}}^{j_{k+1}}\dots \delta _{i_{n}]}^{j_{n}}\,.$

He changed equation 8 to say:

$\varepsilon _{i_{1}\dots i_{k}~i_{k+1}\dots i_{n}}\varepsilon ^{i\dots i_{k}~j_{k+1}\dots j_{n}}=k!~\delta _{[i_{k+1}}^{j_{k+1}}\dots \delta _{i_{n}]}^{j_{n}}\,$

thereby mistakenly removing $(n-k)!\,.$
Notice that according to section Levi-Civita symbol#Notation (supported by article Antisymmetric tensor),

$M_{[ab]}={\frac {1}{2!}}(M_{ab}-M_{ba})\,.$

Thus equation 6 is equivalent to:

$\varepsilon _{ijk}\varepsilon ^{imn}=2~\delta _{[j}^{m}\delta _{k]}^{n}\,$

which is an instance of the correct form of equation 8, not his altered form. JRSpriggs (talk) 14:33, 18 August 2010 (UTC)

Levi- Chivita symbol - lower and upper indicies.

Hey ,

I've fixed an identity : (Epsilon) with lower indicies should be equal to Minus the (Epsilon) with upper indicies. Not as it was mistakenly even specifically stated that "the sign should not change". —Preceding unsigned comment added by 132.68.132.186 (talk) 21:19, 21 November 2010 (UTC)

Sorry guys, I've seen your discution on the matter above just now. I must point that according to what I am told by my prof.s (physics) the Levi- Chivita symbol indeed needs to be treated as a tensor for this matter - I was taught the same proof given above for the sign change, in class. —Preceding unsigned comment added by 132.68.132.186 (talk) 21:35, 21 November 2010 (UTC)

Apparently you and perhaps your professor are confused. I added the subsection Levi-Civita symbol#Ordinary tensor specifically to try to show the difference between what I was talking about regarding tensor density and what others think they are talking about. JRSpriggs (talk) 08:44, 22 November 2010 (UTC)

Again the section on tensor densities

On his talk page, user Xihr (talk · contribs) wrote:

An all-contravariant tensor (density) cannot possibly equal an all-covariant tensor (density). They are simply not the same types of things. It would be like saying that a matrix equals a turnip. Perhaps you meant that the components of the tensors (densities) are equal, but even that doesn't sound right; the relation depends on the metric signature.  Xihr  10:22, 18 November 2011 (UTC)

I will respond here.
Indeed, the components are the same in all coordinate systems. This is because they are both defined to be the Levi-Civita symbol in all coordinate systems. You may ask, how is that possible? According to the article Tensor density, when you transform the contravariant tensor density of weight +1 from one coordinate system to another you do this:

$\varepsilon ^{\alpha \beta \gamma \delta }=\left(\det {\left[{\frac {\partial {\bar {x}}^{\iota }}{\partial {x}^{\omega }}}\right]}\right)^{+1}\,{\frac {\partial {x}^{\alpha }}{\partial {\bar {x}}^{\mu }}}\,{\frac {\partial {x}^{\beta }}{\partial {\bar {x}}^{\nu }}}\,{\frac {\partial {x}^{\gamma }}{\partial {\bar {x}}^{\mathrm {o} }}}\,{\frac {\partial {x}^{\delta }}{\partial {\bar {x}}^{\sigma }}}\,{\bar {\varepsilon }}^{\mu \nu \mathrm {o} \sigma }={\bar {\varepsilon }}^{\alpha \beta \gamma \delta }\,.$

where the first equality is the transformation law for tensor densities and the second equality follows from the properties of the determinant of a matrix. A similar equation can be derived for the covariant tensor density of weight -1, to wit:

$\varepsilon _{\alpha \beta \gamma \delta }=\left(\det {\left[{\frac {\partial {\bar {x}}^{\iota }}{\partial {x}^{\omega }}}\right]}\right)^{-1}\,{\frac {\partial {\bar {x}}^{\mu }}{\partial {x}^{\alpha }}}\,{\frac {\partial {\bar {x}}^{\nu }}{\partial {x}^{\beta }}}\,{\frac {\partial {\bar {x}}^{\mathrm {o} }}{\partial {x}^{\gamma }}}\,{\frac {\partial {\bar {x}}^{\sigma }}{\partial {x}^{\delta }}}\,{\bar {\varepsilon }}_{\mu \nu \mathrm {o} \sigma }={\bar {\varepsilon }}_{\alpha \beta \gamma \delta }\,.$

I hope this clears it up. JRSpriggs (talk) 11:32, 19 November 2011 (UTC)

metric signature

Hi all

This is my first contribution, so apologies if the wiki protocol is not followed to the dot. However I was surprised, and found it a bit rude, to receive no message on my talk page or here explaining why my recent addition of the manifold signatures in the Sec.2 of the article was purely suppressed by JRSpriggs. I was aware that these changes were insufficient as I concentrated on just this section and didn't bother with the proof, as I added the reference to the Wald 84 book. I got involved as I was using this page to check on a calculation, but had a sign error. Later I found in Wald (1984) that the correct n-dimensional eq. (8) of the article should include the signature of the manifold, which was -1 in the Lorentzian case. In the absence of the signature term, the expression only treats Euclidean manifolds, which usually doesn't concern Relativist's spacetime manifolds. Having little time, I changed the places where I felt it mattered most, gave the reference and left it for more motivated contributors to complete according to this extra point the article where necessary. So unless there is a notation subtlety somewhere in the article that escaped me, I don't understand why a correct, yet incomplete addition to the article was not completed but rather purely erased. Please can JRSpriggs answer? I thought putting the debate here was also of interest to others and so did not wrote directly to his talk page. Thanking you in advance yours, sincerely, mpld — Preceding unsigned comment added by Mpld (talk • contribs) 20:45, 26 January 2012 (UTC)

You are mistaken in thinking that the metric is involved in the definition of the Levi-Civita symbol. It can be defined on manifolds which do not even have a metric. In any case, your changes are inconsistent with other parts of the article. You might benefit from reading the other sections of talk above which explain many similar issues.

I am sorry that I could not explain this earlier, but many people make ill-considered edits like yours and I do not have the time to explain all my reversions since I watch over two hundred articles. I usually give explanations only if the other person persists in trying to make his change or asks a question about it on the talk page as you have done here. JRSpriggs (talk) 05:13, 27 January 2012 (UTC)

Notation section?

What is this section doing here? Its all about antisymmetric tensors and has the "main article link", so the entire section will be moved to that article. Just because the formulae make use of the symbol does it mean they necessarily need to be included? No. We can just link from here to there. F = q(E+v×B) ⇄ ∑_ic_i 05:49, 11 April 2012 (UTC)

Please be more specific. I do not see what in this Levi-Civita symbol article you are referring to. — Quondum^☏ 07:56, 11 April 2012 (UTC)

Sorry, I should have been clearer - the subsection was actually called "Notation" at the very end. Its been moved now in this edit: [1]. F = q(E+v×B) ⇄ ∑_ic_i 09:00, 11 April 2012 (UTC)

clean up

Main changes:

1. Re-organize - it was a sort of a mess and repetitive,
2. add missing and needed links,
3. make the notation consistent throughout (this is another article which uses a mix of bold/italics for vectors and matrices, so I'll clarify slightly by using lower case bold for vectors and capital bold for matrices),
4. cleared out a silly amount of inline LaTeX for individual characters which could easily have been typed as html (such as $1$, $i\neq j$, "$i$th component", $3!=6$ etc.) - it doesn't look good having so much of it because of the sudden jumps in font (between Arial and Computer/Latin modern roman) size and style differences. Can understand this for certain things like Dirac notation $|\psi \rangle$ or blackboard bold $\mathbb {R} ^{n}$ (or Greek/maths letters which are at least slightly more clearer in LaTeX than Arial, such as lambda $\lambda$ / λ, tau $\tau$ / τ, or script "ell" $\ell$ / ℓ) that are not as easily obtained any other way, that much is tolerable.

F = q(E+v×B) ⇄ ∑_ic_i 21:58, 25 April 2012 (UTC)

ε or ϵ?

Text of the article currently uses ε (a.k.a. $\varepsilon$), not ϵ. Are you sure that there is nothing wrong with this? Incnis Mrsi (talk) 11:20, 11 January 2013 (UTC)

As far as I know, they are just two different fonts for writing epsilon. Although someone claimed in the text that some authors make a distinction, I am not aware that there is any agreed upon rule to that effect. And I have not tried to follow that in this article because the two versions of the tensor density are numerically identical. What would be the point of having two different symbols for the same thing? JRSpriggs (talk) 16:29, 11 January 2013 (UTC)

IMHO there is no point of having two different symbols for the same thing, hence one of two should be selected to be declared the "correct" (MoS-compliant) notation. "Different fonts" are irrelevant. The word "font", as I can realize from the context, actually assumed a "typeface". Are you sure that understand correctly this concept? Such cases as ε—ϵ and φ—ϕ are actually allography, like ɑ—a, ℓ—l and quite recent ¦—| – it has little to do with typefaces. Different "fonts", of course, may use different allographs, but these are not typefaces which distinguish between allographs. With the advent of Unicode, many allographs diverged to separate code points, as you can see in my samples above. This is not the case for the same letter written in different typefaces, such as I (capital "i" in serif) and I (capital "i" in sans). Incnis Mrsi (talk) 18:54, 11 January 2013 (UTC).

Looking at the first few Google hits for "Levi-Civita tensor", most seem to use "ϵ". Some use "ε". One used them both interchangeably. One used "ϵ" for the ordinary tensor which I called "E" here, and "[]" (with the indices between the brackets) for the tensor density. So there appears to be a lack of consistency.

Although you feel that these two symbols "ε" and "ϵ" should be regarded as different characters, to me they are both just epsilon (drawn in different styles). JRSpriggs (talk) 05:56, 12 January 2013 (UTC)

It was me who made the claim about using the two as distinct symbols, and I should have stated it as "at least one author". Upon review, I am in favour of removing this claim entirely as being non-notable and of little value. It probably does not make sense in this context to stress any distinction between the typographic variants, rather only to use one of them consistently within the article. Though I do not agree that the two tensor densities are "the same thing", within the framework of tensor densities there is no harm in using the same symbol in this case. So I am in general agreement. — Quondum 07:32, 12 January 2013 (UTC)

Ordinary tensor

"One of these ordinary tensor fields may be converted to the other by raising or lowering the indices with the metric as is usual, but a minus sign is needed if the metric signature contains an odd number of negatives."

It's might be more complicated than this. If the spacetime dimension d is odd, then the number of negatives in the metric is either 4,6,... (even) or 1 (odd) depending on the choice of metric signature, e.g. (+----) or (-++++) for d = 5.

In addition, with the choice (-++++...) for d odd, E is actually a tensor (even under parity inversion, i.e. not a pseudo-tensor). See Weinberg, The Quantum Theory of Fields vol.1. I'm not very sure about these matters myself. YohanN7 (talk) 17:31, 26 February 2013 (UTC)

Could you give a more specific example of what you think is wrong with that section of the article?

As far as I can make sense of what you are saying, it does not contradict what that section says. In particular, the section does not claim that E is a pseudo-tensor. JRSpriggs (talk) 06:21, 27 February 2013 (UTC)

I didn't say anything is wrong. I am opening for the possibility that some clarification is needed. For instance, just because the section does not claim that E is a pseudo-tensor, doesn't exclude the possibility of having the text say that E may be a tensor under certain conditions. In particular, for spacetimes in dimension D = d + 1, do you presuppose the minus convention (mostly minuses) for the metric, the plus convention (mostly plus) or neither?

• If plus convention, then, according to the text, there is always minus-sign because there is exactly one minus in the metric.
• If minus convention, then, according to the text, there is a minus signs only if the dimension d of space is odd.
• If neither, then, according to the text, one of the above apply according to the choice of metric convention.

To put the question clearer: Is it sufficient to count minuses in my metric to deduce the presence of a minus sign? Also, do you have a source for the statements and equations in that section?

Even clearer: Does the tensor or pseudotensor character of E in a given spacetime depend on the metric convention? YohanN7 (talk) 13:00, 27 February 2013 (UTC)

What about the formulas I mention in the above topic? To be clear about my problem, given the formulas in the article I don't understand how to expand the product. (It is not clear how to use the upper product index n, and it is far from clear how the factors ⁠1/2⁠, ⁠1/12⁠, ... come about when using the first two or the last formula. The third formula is "almost" clear, only the upper product index n is ambiguous.)

I suggest that the lead includes mention of several different objects named the Levi-Civita symbol. The reason is that the alternative (but very common) descriptions appear at the end of the very end article. YohanN7 (talk) 15:15, 27 February 2013 (UTC)

Levi-Civita symbol#Ordinary tensor makes no presupposition regarding the number of minus signs in the signature (except for the example of Minkowski space which is assumed to have either 1 or 3 minus signs).

As the section says, "a minus sign is needed if the metric signature contains an odd number of negatives".

As the section says, both the contravariant E and the covariant E are ordinary tensors. Thus they are never pseudo-tensors.

I suggest that you ignore the formulas which you were asking about in the previous section of talk. They are not helpful, but merely cause confusion for most people. The useful fact is that the Levi-Civita symbol is: positive if the values of the indices are an even permutation of (1,2,...n), negative if they are an odd permutation, and zero if any index is repeated. For the tensor densities $\varepsilon \,,$ the absolute value is always 1 or 0. For the ordinary tensors E, the absolute value is either ${\sqrt {\vert g\vert }}\,$ (if covariant) or its reciprocal (if contravariant) or 0. JRSpriggs (talk) 01:00, 28 February 2013 (UTC)

On second thought, the E have an extra sign change under orientation reversing coordinate transformations. So they are always pseudo-tensors. I forgot that the product of two such pseudo-tensors could be an ordinary tensor and thus fulfill the necessary equations.

What bothers me now is that pseudotensor is ambiguous terminology. It could just indicate one additional sign change under an orientation reversing coordinate transformation or it could be much more pathological such as the Stress–energy–momentum pseudotensor or even worse the Christoffel symbols. So I am not sure what is the best way to reword the section. JRSpriggs (talk) 02:41, 28 February 2013 (UTC)

Thank you for the clarification!

• For the ambiguity in the term pseudotensor, I suggest "pseudotensor"->"pseudotensor in the sense of Lorentz the appropriate O(p,q) transformation properties" at first mention.
• At least my problems with the formulas are resolved provided the upper product limit n is replaced by the condition i,j ≤ n. Originally, I mistook the n for the number of factors in the product (which is why I worked them out as in the previous topic to begin with). One might write:

$\varepsilon _{a_{1}a_{2}a_{3}\ldots a_{n}}=\operatorname {sgn} \!\left(\prod _{i<j,i,j\leq n}(a_{j}-a_{i})\right)=\mathrm {sgn} (a_{2}-a_{1})\mathrm {sgn} (a_{3}-a_{1})\cdots \mathrm {sgn} (a_{n}-a_{1})\mathrm {sgn} (a_{3}-a_{2})\cdots \mathrm {sgn} (a_{n}-a_{n-1})$

and the like for the others and they become as clear as a day.

• "On second thought, the E have an extra sign change under orientation reversing coordinate transformations." This is something I don't understand. I trust you, but Weinberg writes "... odd-dimensional spacetime, because here the the tensor ϵ is even [my emphasis] under the inversion of space coordinates.", and I trust him as well. (He is using the (-,+,+,+,...) metric.) I'll simply have to dig into the details.YohanN7 (talk) 04:37, 28 February 2013 (UTC) YohanN7 (talk) 18:20, 28 February 2013 (UTC)

I remember now that, in writing Levi-Civita symbol#Tensor density, I was trying to avoid delving into the very messy distinctions among: authentic tensor densities, pseudo tensor densities, even tensor densities, and odd tensor densities. These are defined at Tensor density#Definition.

As I explained above at #Again the section on tensor densities, the $\varepsilon \,$ are authentic tensor densities, that is, their transformation law involves the determinant of the coordinate transformation without any absolute value or sgn functions. Since their weight is +1 (if contravariant) or −1 (if covariant), they may also be described as odd.
Notice that I referred above to "orientation reversing transformations" rather than "inversions" because in an even dimensional space an inversion (meaning all coordinates are negated) is not orientation reversing.
I think that I shall change "ordinary" to "absolute" which merely means that the weight is zero without specifying whether the tensor is authentic (even) or pseudo (odd). JRSpriggs (talk) 10:22, 2 March 2013 (UTC)

Explanation (08/05/2013)

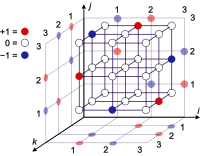
Sorry, tried posting this earlier only to loose the internet connection for a while... For this edit, the caption did absent-mindedly refer to repeated indices which do not form permutations and I intended to correct it to:

For the indices i, j, k in ε_ijk,

cyclic permutations (yellow) are ijk, jki, kij corresponding to ε = +1, while

anticyclic permutations (red) are ikj, kji, jik corresponding to ε = −1,

otherwise ε = 0.

This particular diagram is not absolutely essential - it was hoped to pictorially show the cyclic/anticyclic permutations. Feel free to delete even the present one by all means.

About "why duplicate an existing diagram and remove another?", the previous diagram

shows a left-handed coordinate system, so why not draw another one similar to it:

Left-handed

to match:

Right-handed

? I have no intention to "dominate" or "dismiss" the author (user:Belgariath) of the former one with cubes and hollow shading (file:LeviCivitaTensor.jpg), however:

1. it is in JPG when diagrams of this type can and should be in SVG,
2. less clear/cleaner/aesthetic to user:Qniemiec's style of design.

Hope that clarifies things. M∧Ŝc²ħεИ_τlk 14:22, 8 May 2013 (UTC)

I did not notice that you had changed the handedness of the coordinates. It was not my intent to have a left-handed diagram. Indeed making them all right-handed would probably be better.

So go ahead and try again to do your edit with the caption improvement. JRSpriggs (talk) 14:48, 8 May 2013 (UTC)

I went ahead and replaced the permutation diagram but left file:LeviCivitaTensor.jpg. I think it should just be removed, but to prevent becoming a gatekeeper of images in this article I'll leave that for someone else. M∧Ŝc²ħεИ_τlk 15:30, 8 May 2013 (UTC)

I find the references to "left-handed" and "right-handed" here make me do a double-take. The seems to refer to the representation of the index values, not to the coordinates of the space. To even mention handedness in this context seems to me to be inappropriate and ambiguous. Also, we have three equivalent diagrams; shouldn't we remove two of them? — Quondum 02:09, 9 May 2013 (UTC)

Despite the incorrect name, file:Epsilontensor.svg looks nice to me. So does file:Levi-Civita Symbol cen.svg. But as I said, anyone is free to delete what images they like. M∧Ŝc²ħεИ_τlk 04:29, 9 May 2013 (UTC)

I think all of the "3D" diagrams are hard to read. Perhaps having it done three different ways gives the reader a better chance to find one of which he/she can make sense. JRSpriggs (talk) 05:36, 9 May 2013 (UTC)

Fair point actually. M∧Ŝc²ħεИ_τlk 05:51, 9 May 2013 (UTC)

I agree with JSpriggs that the three diagrams are hard to read. I fact, since they don't even have mnemonic value, I fail to see any value in them. The other diagram (File:Permutation_indices_3d.svg) gets to communicate something useful, but it is not correct as it stands. It is permutations of concrete indices (1, 2, 3) that are relevant to specific values of ε, not permutations of the abstract indices (i, j, k). — Quondum 15:51, 14 May 2013 (UTC)

PS: Since the three diagrams are representing values of matrices, the only diagram that is immediately interpretable as a 3rd-order matrix is File:Epsilontensor.svg, so I'll remove the other two from the article. — Quondum 15:59, 14 May 2013 (UTC)

Permutation indices 3d numerical

Actually Quondum File:Permutation_indices_3d.svg is correct, since you can take cyclic permutations of the indices without substituting for numbers:

$\varepsilon _{ijk}=\varepsilon _{jki}=\varepsilon _{kij}=-\varepsilon _{ikj}=-\varepsilon _{jik}=-\varepsilon _{kji}$

Anyway, if you require, I created a numerical version, feel free to substitute. M∧Ŝc²ħεИ_τlk 17:22, 14 May 2013 (UTC)

The relations you give here are true for any values of i, j and k. However the relations which give specific values to the Levi-Civita symbols (as in the cyclic illustration) are true only for specific values of these indices, not in general. Hence, the diagram is not valid for arbitrary indices i, j and k. Another tweak: I'd suggest that the symbols in the centre of the cycles would be more appropriately be +1 and −1 and not + and −, since the diagram deals with the components of the Levi-Civita symbol (I know this does not look as pretty, but the semantics should be as clear as possible). — Quondum 23:47, 14 May 2013 (UTC)

Fixed. M∧Ŝc²ħεИ_τlk 06:54, 15 May 2013 (UTC)

Thanks, I've updated the article with this. — Quondum 11:24, 15 May 2013 (UTC)

Cross product

Should we emphasize in the introduction, what's this symbol primarily is used for?

I think the main reason one would put this symbol into his equations is to express a cross product, or more generally to express an n dimensional vector that's perpendicular/orthogonal to another n-1 of n dimensional vectors (and the related stuff coming from this).

Calmarius (talk) 12:44, 31 August 2013 (UTC)

Do you have a reliable source for the statement that this is the principal use of the Levi-Civita symbol? It might be so in your experience, but perhaps not for others. JRSpriggs (talk) 11:00, 1 September 2013 (UTC)

Calmarius: The Levi-Civita symbol ties a number of things together: expressing determinants in index form, components of elements of the exterior algebra (i.e. the exterior product of p vectors to obtain a p-vector, or exterior product of p one forms to obtain a p-form), and it seems you're referring to Hodge duality?

This article really needs a decent lead to overview what the symbol is for, and a history section. M∧Ŝc²ħεИ_τlk 20:56, 1 September 2013 (UTC)

Lead

After all that, not sure if the lead is adequate as we jump into tensor densities and vector spaces... but its very hard to suddenly use index notation without saying what the number of indices corresponds to (a reader would think "why are there n indices?"), and we need to say what the LC symbol is from the very beginning and not pretend it's something else simpler (only to go on later and say it's a tensor density). I tried to tie together the things mentioned in this thread: cross and exterior products, determinants, parallelotopes and their hypervolumes, Hodge duality...

The lead is trickier than I thought... M∧Ŝc²ħεИ_τlk 10:31, 2 September 2013 (UTC)

Yes, the lead does need serious work. Most of its current content must be moved into the body; perhaps we should move that into a "Description" section first and then make the lead into a brief outline. After that, the "Description" can be reworked into "History", "Definition", "Properties", etc. Its fields of use and other names should be mentioned at the start. I would expect the only properties that need be mentioned in the lead are that it is essentially a function on n parameters (n can be chosen to take any value), which each may take the integer values 1 to n, and that it takes the values +1, −1 or 0 respectively if the values of its parameters is an even permutation, odd permutation or not a permutation of the sequence 1, ..., n. Its typical notation as a subscripted epsilon can be mentioned, without mentioning tensor notation in the lead. (You may note that the definition I've given does not allude to vector spaces, linear algebra, tensors, pseudo-tensors etc.) A mention of its uses in tensor analysis, expressing determinants, tensor densities, as a cross product in 3d etc. risks immediately cluttering the lead excessively, and these aspects can be dealt with at length in the article. Strictly speaking, it does not even determine an orientation in a vector space. The current statement that it represents orthogonality is incorrect: it is defined in the complete absence of a metric, without which orthogonality is utterly undefined (even though the LC symbol is usually not much use in the geometric setting unless the scale and orientation of volume element is determined, so it would be most useful specifically with an oriented pseudo-orthonormal basis). I think we should avoid giving it a tensor-centric interpretation in the lead, as well as avoiding giving this approach too much weight in the article. — Quondum 12:49, 2 September 2013 (UTC)

I'll get back to this later, but there doesn't seem to be much point moving bits into the article, instead the lead should just be trimmed/rewritten. M∧Ŝc²ħεИ_τlk 12:58, 2 September 2013 (UTC)

BTW, yes, I know the LC symbol is independent of a metric, and although "can be used to represent orthogonality" is badly written, it's not immediately wrong - the cross product of two vectors in 3d is perpendicular to the exterior product, the two are related by the Levi-Civita symbol. M∧Ŝc²ħεИ_τlk 15:31, 2 September 2013 (UTC)

Done for now. The lead is as good as I can make it... Others will have to edit for themselves, but suggestions are of course welcome here. M∧Ŝc²ħεИ_τlk 19:20, 2 September 2013 (UTC)

That was a substantial improvement, thanks.

On "the cross product of two vectors in 3d is perpendicular to the exterior product, the two are related by the Levi-Civita symbol": no, this is not so, it is only true for 3d orthogonal right-handed basis. The orientation of the space comes from the handedness of the basis you choose, and the dependence of the cross product on the metric is introduced via the restriction to an orthonormal basis. Though given two vectors, it will generate a covector that is orthogonal to each of the input vectors in the sense of a biorthogonal system (but not in the sense of a bilinear form), but is nevertheless still of undefined length. — Quondum 03:12, 3 September 2013 (UTC)

Formulas

Some generalized formulae are:

$\varepsilon _{a_{1}a_{2}a_{3}\ldots a_{n}}=\operatorname {sgn} \!\left(\prod _{i<j}^{n}(a_{j}-a_{i})\right)=\ldots$

where n is the dimension (rank),...

Is this the same as

$\varepsilon _{a_{1}a_{2}a_{3}\ldots a_{n}}={\frac {1}{0!\cdots (n-1)!}}\left(\prod _{i<j,i,j\leq n}(a_{j}-a_{i})\right)=\ldots ?$

In the case n = 3 the latter becomes, with a₁ ↔ i, a₂ ↔ j, and a₃ ↔ k,

$\varepsilon _{ijk}={\frac {1}{1*1*2}}\left(\prod _{i<j,i,j\leq 3}(a_{j}-a_{i})\right)={\frac {1}{2}}(j-i)(k-i)(k-j),$

and the with n = 3 one obtains

$\varepsilon _{ijk}={\frac {1}{12}}(j-i)(k-i)(k-j)(l-i)(l-j)(l-k).$

These expressions match the earlier formulas for n = 2 and n = 3. They also match the succeeding formula provided the latter's upper index in the product is interpreted to mean i,j≤n.

The far right formulas probably need some interpretation too, but I haven't attempted do decipher these. YohanN7 (talk) 16:40, 26 February 2013 (UTC)

Do you have a reference for the formula you propose? Signatures of permutations can be a pain to determine from adjacent transpositions of indices every time so it would be a good addition. M∧Ŝc²ħεИ_τlk 16:53, 26 February 2013 (UTC)

No reference I'm afraid, but none of the formulas in the article makes much sense to me. YohanN7 (talk) 17:55, 26 February 2013 (UTC)

For the record - one of the formulae already in the article:

$\varepsilon _{a_{1}a_{2}a_{3}\ldots a_{n}}={\frac {1}{G(n+1)}}\prod _{i<j}^{n}(a_{j}-a_{i})=\prod _{i=1}^{n-1}\left({\frac {1}{i!}}\,\prod _{j=i+1}^{n}(a_{j}-a_{i})\right)$

is exactly as the same as the one YohanN7 gives above, just written in an obscure form. I rewrote the segment to simplify, better? Unfortunately I haven't found a source for this equation (Schaum's Tensor calculus by Kay gives a restricted case for all indices unequal), anyway that can be added any time. M∧Ŝc²ħεИ_τlk 00:36, 4 September 2013 (UTC)

Zero and 1d symbols?

I have never come across these before, and are they ever needed? For the 1d case, considering determinant of a 1×1 matrix, is it just 1? What would be the zero dimensional case? Sources? Given their definitions, they could be mentioned somewhere in the article. They would have to be separately defined, since only one permutation is possible (0! = 1! = 1), although there is no antisymmetry. M∧Ŝc²ħεИ_τlk 00:36, 4 September 2013 (UTC)

I doubt that you'll find notability, my edit comment related to avoiding an invalid implication. So I removed the statement that the 2d version was the "simplest", a completely unnecessary statement. I'm not suggesting includng a mention of the 0d and 1d cases in the article though. Yes, the Levi-Civita symbol in one dimension would consist of 1¹ = 1 value, with 1! = 0 1! = 1 nonzero value, which is +1, which for the determinant multiplies the only element of a 1×1 matrix. The zero-dimensional case is consistent with the definitions, despite having no practical value: there are 0⁰ = 1 value, with 0! = 1 nonzero value, which is +1 (but no indices: a scalar multiplier). The determinant of every 0×0 matrix is 1. And on antisymmetry: no, every order-0 and order-1 tensor is totally antisymmetric – vacuously so. — Quondum 01:08, 4 September 2013 (UTC)

So basically:

$\varepsilon =1\,,\quad \varepsilon _{1}=1$

for 0d and 1d respectively? For completeness it would be nice if this was included somewhere. For the 2d symbol, I should have said it's the simplest non-trivial example. M∧Ŝc²ħεИ_τlk 09:12, 4 September 2013 (UTC)

Yes, you have those correct by my reckoning. I also think that mentioning them would be nice (I'm a big fan of including the trivial cases in any general definition), though I am not about to argue if someone claims that they are OR or not notable. I see trivial cases being excluded regularly, for no apparent reason differnt from that which applied historically for the exclusion of zero as a number: its behaviour is so simple that one often doesn't realize that it is there and that it fits a regular structure, and so it confuses people. So they chop holes in every structure and theorem so that they do not have to consider it. In a reference, however, it makes sense to address these cases.

I had considered exactly what you put here: saying that it is the simplest nontrivial case. However, I came to the conclusion that to mention "simplest" at all in this context would be inappropriate: it is stating the obvious pointlessly, and is borderline editorializing. — Quondum 16:18, 4 September 2013 (UTC)

Completeness is always nice, although what I don't like is that we're forced to say "vacuously totally antisymmetric" which is really confusing, and without a source it just looks like OR... For the space it takes to include this, it may be of very little value for most readers. Feel free to add this yourself to the definitions section. M∧Ŝc²ħεИ_τlk 14:17, 5 September 2013 (UTC)

I don't think that we need to (or shouild) say it – it's fine as it stands. If we find that the n = 0 and n = 1 cases are queried, then we could explain any potentially confusing detail such as this in a footnote. — Quondum 21:13, 5 September 2013 (UTC)

questions about equation 4 ($\varepsilon _{ijk}\varepsilon ^{imn}=\delta _{j}{}^{m}\delta _{k}{}^{n}-\delta _{j}{}^{n}\delta _{k}{}^{m}$)

1) Is this meant to be in Einstein notation? I ask because if it is a simple product rather than the sum of products

$\varepsilon _{112}\varepsilon ^{112}$ = 0x0 = 0, but

$\delta _{1}{}^{1}\delta _{2}{}^{2}-\delta _{1}{}^{2}\delta _{2}{}^{1}$ = 1x1 - 0x0 = 1.

2) Why are there proofs of equations 1,2,3,5,6 but not 4? --Wikimedes (talk) 05:35, 28 November 2013 (UTC)

The equation in the title just above is in Einstein summation notation, not a product of tensor and pseudotensor components:

$\varepsilon _{ijk}\varepsilon ^{imn}\equiv \sum _{i=1,2,3}\varepsilon _{ijk}\varepsilon ^{imn}\equiv \varepsilon _{1jk}\varepsilon ^{1mn}+\varepsilon _{2jk}\varepsilon ^{2mn}+\varepsilon _{3jk}\varepsilon ^{3mn}$

then you choose j, k, m, n and then substitute for values of the LC symbol and delta.

About proofs, I have no opinions or comments. M∧Ŝc²ħεИ_τlk 10:58, 28 November 2013 (UTC)

Formula 4 is proved a bit higher up than the others. YohanN7 (talk) 15:29, 28 November 2013 (UTC)

A yes, a special case of the determinant. Thanks.--Wikimedes (talk) 20:01, 28 November 2013 (UTC)

Thanks. I made a note in the article that Einstein notation is being used.--Wikimedes (talk) 20:01, 28 November 2013 (UTC)

Tensor section

I made a change to the Levi-Civita tensor section a couple of weeks ago which was reverted without an explanation. So I figured I'd explain my reasoning here in the hopes that my edit will be kept.

As a working theoretical physicist (personal webpage) I found the convention used in previous versions to be unnecessarily confusing and contrary to what's used throughout the field today. If you have two tensors with the same symbol, one with upper indices and one with lower, it's universally understood that they're related by raising/lowering with the metric tensor. When doing calculations in theoretical physics one generally doesn't even think about this - indices are freely raised and lowered as needed. In the earlier versions of this page, however, the upper- and lower-index Levi-Civita tensors were related to each other by an overall minus sign. This would strike any reasonable physicist as extraordinarily silly, and would, without a doubt, lead to sign errors propagating in any calculation which uses this page as a reference.

This isn't just a quirk of mine: for example, the entire presentation I wrote in the tensor densities section closely follows Carroll's GR book, which is as standard a modern text on the subject as there is.

I strongly recommend keeping the convention in there so as to avoid confusing readers for absolutely no reason. — Preceding unsigned comment added by 128.91.41.35 (talk) 22:07, 19 January 2017 (UTC)