File:Uniaxial.png
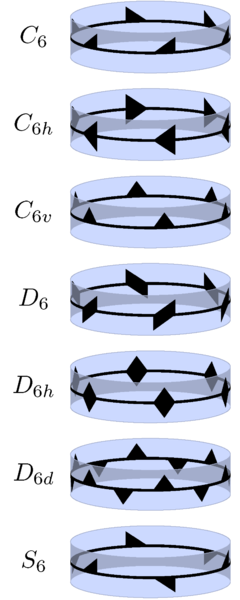
Size of this preview: 240 × 600 pixels. Other resolutions: 96 × 240 pixels | 192 × 480 pixels.
Original file (800 × 2,000 pixels, file size: 26 KB, MIME type: image/png)
 | This is a file from the Wikimedia Commons. Information from its description page there is shown below. Commons is a freely licensed media file repository. You can help. |
|
This diagram image could be re-created using vector graphics as an SVG file. This has several advantages; see Commons:Media for cleanup for more information. If an SVG form of this image is available, please upload it and afterwards replace this template with
{{vector version available|new image name}}.
It is recommended to name the SVG file “Uniaxial.svg”—then the template Vector version available (or Vva) does not need the new image name parameter. |
Summary
- Author: en:user:AndrewKepert
- Toolchain: MetaPost and TeX.
- Source: below
- Description: Illustration of a typical member of each of 7 infinite families of 3D point groups.
- Destination: en:Point groups in three dimensions.
- Permission: GFDL / CC
Source code
Instructions: on a system with a modern TeTeX or similar installed save the following two files, then run
mpost uniaxial && pdftex uniaxial
You will then need to use ghostscript or similar to make a raster image out of the pdf.
Source code author: en:user:AndrewKepert
Source code license: GPL
Source code
PostScript code
picture pic[];
pair pt[],pt[]n,pt[]e,pt[]w,pt[]s,pt[]ne,pt[]nw,pt[]se,pt[]sw;
pair ux,uy,uz;
path unitcircle; unitcircle=fullcircle scaled 2;
boolean front[];
color colour[];
path p[];
u=16;
ux=.4*down*u;
uy=right*2u;
uz=up*.5u;
transform xyplane[];
(0,0) transformed xyplane0 = (0,0);
(1,0) transformed xyplane0 = ux;
(0,1) transformed xyplane0 = uy;
for i = -1 step 1/16 until 1:
xyplane[i]=xyplane[0] shifted (i*uz);
endfor
theta=10;
alpha=8;
N:=6;
for i = -1 step .5 until N+1:
pt[i] = right rotated theta rotated (360i/N) transformed xyplane0;
front[i]= ypart pt[i] < ypart xyplane0;
pt[i]e = right rotated (theta+alpha) rotated (360i/N) transformed xyplane0;
pt[i]w = right rotated (theta-alpha) rotated (360i/N) transformed xyplane0;
pt[i]n = right rotated theta rotated (360i/N) transformed xyplane[.75];
pt[i]ne = right rotated (theta+alpha) rotated (360i/N) transformed xyplane[.75];
pt[i]nw = right rotated (theta-alpha) rotated (360i/N) transformed xyplane[.75];
pt[i]s = right rotated theta rotated (360i/N) transformed xyplane[-.75];
pt[i]se = right rotated (theta+alpha) rotated (360i/N) transformed xyplane[-.75];
pt[i]sw = right rotated (theta-alpha) rotated (360i/N) transformed xyplane[-.75];
endfor
t0=directiontime uz of (unitcircle transformed xyplane0);
t1=directiontime -uz of (unitcircle transformed xyplane0);
t2=t0+length unitcircle;
path backface,frontface;
backface:=(subpath (t0,t1) of unitcircle transformed xyplane[1])
-- (subpath (t1,t0) of unitcircle transformed xyplane[-1])
-- cycle;
frontface:= (subpath (t1,t2) of unitcircle transformed xyplane[1])
-- (subpath (t2,t1) of unitcircle transformed xyplane[-1])
-- cycle;
colour0:=(.8,.85,1);
colour1:=.8[black,colour0];
colour2:=.6[black,colour1];
def constructribbon(expr delta)=
% stuff on back face
pic1:=image( for i = 0 step delta until N-eps: if not front[i]: fill p[i]; fi endfor
fill (subpath (t0,t1) of unitcircle transformed xyplane[1/16])
-- (subpath (t1,t0) of unitcircle transformed xyplane[-1/16])
-- cycle;);
% stuff on front face
pic2:=image( for i = 0 step delta until N-eps: if front[i]: fill p[i]; fi endfor
fill (subpath (t1,t2) of unitcircle transformed xyplane[1/16])
-- (subpath (t2,t1) of unitcircle transformed xyplane[-1/16])
-- cycle;);
% all of back face
pic0:=image(fill frontface withcolor colour0;
fill backface withcolor colour1;
draw pic1 withcolor colour2);
fill backface withcolor colour0;
fill frontface withcolor colour0;
draw pic1;
clip pic0 to frontface;
draw pic0;
draw pic2;
draw unitcircle transformed xyplane[1] withpen pencircle scaled 0.2 withcolor colour1;
draw subpath (t2,t1) of unitcircle transformed xyplane[-1] withpen pencircle scaled 0.2 withcolor colour1;
enddef;
beginfig(1)
for i=0 upto N-1:
p[i]:= pt[i]--pt[i]w--pt[i]ne--pt[i]e--cycle;
endfor
constructribbon(1);
endfig;
beginfig(2)
for i=0 upto N-1:
p[i]:= pt[i]w--pt[i]ne--pt[i]se--cycle ;
endfor
constructribbon(1);
endfig;
beginfig(3)
for i=0 upto N-1:
p[i]:= pt[i]--pt[i]e--pt[i]n--pt[i]w--cycle ;
endfor
constructribbon(1);
endfig;
beginfig(4)
for i=0 upto N-1:
%p[i]:= pt[i]--pt[i]ne--pt[i]e--pt[i]--pt[i]sw--pt[i]w--cycle ;
p[i]:= pt[i]ne--pt[i]e-- pt[i]sw--pt[i]w--cycle ;
endfor
constructribbon(1);
endfig;
beginfig(5)
for i=0 upto N-1:
p[i]:= pt[i]n--pt[i]e--pt[i]s--pt[i]w--cycle ;
endfor
constructribbon(1);
endfig;
beginfig(6)
for i=0 upto N-1:
p[i]:= pt[i]--pt[i]e--pt[i]n--pt[i]w--cycle ;
p[i+.5]:= pt[i+.5]--pt[i+.5]e--pt[i+.5]s--pt[i+.5]w--cycle ;
endfor
constructribbon(1/2);
endfig;
beginfig(7)
for i=0 upto N-1:
if odd i:
p[i]:= pt[i]--pt[i]w--pt[i]ne--pt[i]e--cycle;
else:
p[i]:= pt[i]--pt[i]w--pt[i]se--pt[i]e--cycle;
fi
endfor
constructribbon(1);
endfig;
bye
Data
\input supp-pdf
{\tabskip=5pt \lineskiplimit=5pt \lineskip=\lineskiplimit
\halign{\hfil#\hfil&\hfil$\vcenter{\convertMPtoPDF{#}{1}{1}}$\hfil\cr
$C_6$&uniaxial.1\cr
$C_{6h}$&uniaxial.2\cr
$C_{6v}$&uniaxial.3\cr
$D_6$&uniaxial.4\cr
$D_{6h}$&uniaxial.5\cr
$D_{6d}$&uniaxial.6\cr
$S_6$&uniaxial.7\cr
}
}
\bye
Licensing
I, the copyright holder of this work, hereby publish it under the following licenses:
|
Permission is granted to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or any later version published by the Free Software Foundation; with no Invariant Sections, no Front-Cover Texts, and no Back-Cover Texts. A copy of the license is included in the section entitled GNU Free Documentation License. |
  
|
This file is licensed under the Creative Commons Attribution-Share Alike 3.0 Unported license. | |
| ||
| This licensing tag was added to this file as part of the GFDL licensing update. |
This file is licensed under the Creative Commons Attribution-Share Alike 2.5 Generic, 2.0 Generic and 1.0 Generic license.
- You are free:
- to share – to copy, distribute and transmit the work
- to remix – to adapt the work
- Under the following conditions:
- attribution – You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
- share alike – If you remix, transform, or build upon the material, you must distribute your contributions under the same or compatible license as the original.
You may select the license of your choice.
File history
Click on a date/time to view the file as it appeared at that time.
| Date/Time | Thumbnail | Dimensions | User | Comment | |
|---|---|---|---|---|---|
| current | 08:28, 5 July 2006 | | 800 × 2,000 (26 KB) | AndrewKepert~commonswiki | Author: user:en:AndrewKepert Toolchain: MetaPost and TeX. Source: will be uploaded Description: Illustration of a typical member of each of 7 infinite families of 3D point groups. Destination: en:Point groups in three dimensions. Permission: GF |
File usage
The following page uses this file:
Global file usage
The following other wikis use this file:
- Usage on es.wikipedia.org
- Usage on id.wikipedia.org
- Usage on nl.wikipedia.org
- Usage on ru.wikipedia.org
- Usage on zh.wikipedia.org
